pseudoramble

I am not fantastic at math. I've always found it interesting, but it's never come to me naturally. Sometimes it's good to face things you're not good at and give them a shot! So here we are, goofing off with prime numbers.

So first off, what is a prime number anyway?

Using a grade school explanation of it, prime numbers are natural numbers that can be evenly divided by two divisors – the number itself and 1.

A few things:

• By natural number, I mean whole number (no decimals or complex numbers or crazy things) > 0.
• By divisors, I mean a denominator. So in 10 / 2 = 5, it would be 2.
• Those factors are the number itself (n lets say) and the number 1.

Okay, cool. So some number, itself, and 1. Got it.

What are some prime numbers?

A small sample would be {2, 3, 5, 7}.

How did you figure that out?

Let's first try out a real quick test for prime numbers between 1 and 10:

1. ✘ Trick question. One isn't considered a prime number.

2. ✓ That's our only option here!

3. ✓ We can skip 3 / 3 and 3 / 2 = 1.5 doesn't work. So count 3!

4. ✘ 4 / 2 = 2 so we can't count 4.

5. ✓ 5 / 4 | 5 / 3 | 5 / 2 don't give us whole numbers. So count 5!

6. ✘ 6 / 5 | 6 / 4 won't work. 6 / 3 = 2 so we can't count 6.

7. ✓ 7 / 6 | 7 / 5 | 7 / 4 | 7 / 3 | 7 / 2 don't give us whole numbers. So count 7!

8. ✘ 8 / 4 = 2 so we can't count 8.

9. ✘ 9 / 3 = 3 so we can't count 9.

10. ✘ 10 / 5 = 2 so we can't count 10.

Putting it together, you get the set {2, 3, 5, 7}.

You might notice a few things too:

• All of the even numbers are omitted (save for 2). So right away you knocked out 4, 6, 8, and 10.
• All of the numbers that are multiples of 3 were removed (save for 3). So you could eliminate 6 and 9.
• Even if we somehow missed that 10 / 2 = 5, we would catch that 10 / 5 = 2.
  • In other words, multiples of 5 disappear.

You're finding all numbers that are multiples of something else. Once you've eliminated those numbers, you're left with the prime numbers! And that is the essence of the Sieve of Eratosthenes.

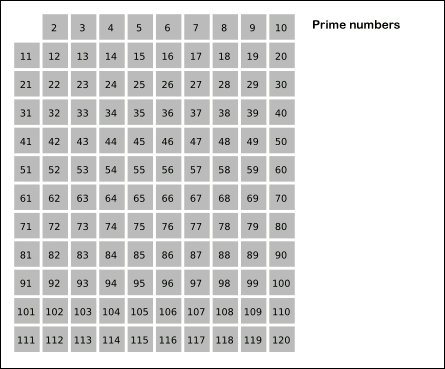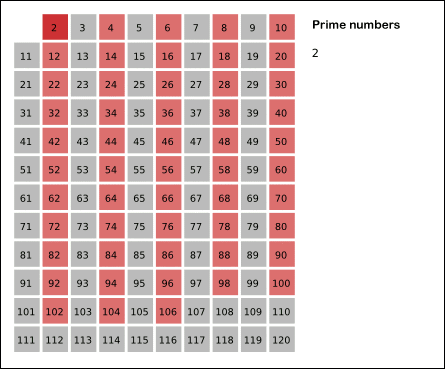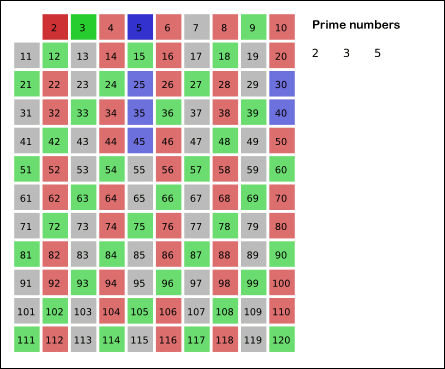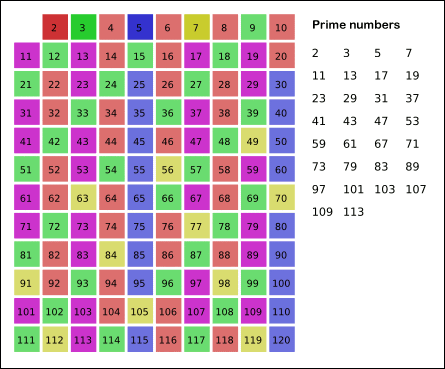
Here's a handy illistration from Wikipedia:

So how do I find the prime numbers under 123,456?!

Running this by hand (or most algorithms really) can be tedious. Good thing we've got these shiny fancy computer boxes now. They're even internet-enabled now!

An implementation of this I put together in F# can be found here. The way I put together my implementation was probably not the most efficient, but I think is somewhat straight forward (here's a paired down form to show the idea):

let rec runTest multipliers limit removals =
    let multipler = List.head multipliers

    if multipler > int (sqrt (float limit))
    then
        removals
    else
        let updatedRemovals = generateStep multipler limit removals
        runTest (List.tail multipliers) limit updatedRemovals

let n = 123456
let startSeq = seq { for i in 2 .. n -> i }

runTest (List.ofSeq startSeq) n Set.empty
|> Set.diffeernce (Set.ofSeq startSeq)

A quick breakdown of the code above:

1. First, check if we need to calculate more primes by looking at the next multiplier. 2. If we do need to check, we generate the values from multiplier^2 to the upper bound specified. 3. We that set of values with the previously calculated value to omit (see Set.union). 4. Repeat from #1.

2. Once here, all the composite numbers have been found. 6. These are removed from the range from 2 to the upper bound (see Set.difference).

3. You are now left with only prime numbers!

So there you have it – A way to find prime numbers. In Part 2, we'll goof around more by talking about how prime numbers actually can make any other natural number.